Analysis of Optimal Polarization State Sampling

Frequency Applied to Photoelastic Analyzer

Chih-Chung Yang

, Chih-Ning Hsu

, Yi-Yung Chen

, Yu-Hsuan Lin

, Hsin-Yi Tsai

Kuo-Cheng Huang

and Chun-Han Chou

National Applied Research Laboratories, Taiwan Instrument Research Institute, 20 R&D Rd. VI,

Hsinchu Science Park, Hsinchu City 30076, Taiwan

Graduate Institute of Color & Illumination Technology,

National Taiwan University of Science and Technology, Taipei City 106335, Taiwan

Keywords: Photoelasticity, Stress, Phase Shift, Doublet Lens.

Abstract: In the paper, we proposed a low-cost photoelastic analysis system and used this system to measure the stress

distribution of the doublet lens. The doublet lens was measured by four types of sampling frequency. The

sampling frequency was 45 degrees, 22.5degrees, 11.25degrees and 5degrees. The different sampling

frequency data was used to build a chart which was the relationship between measurement error and sampling

frequency. The chart could help who used the method to choose a suitable sampling frequency and establish

the most efficient process of stress measurement. In the future, we hope to improve the photoelastic analysis

system to be fully automatic and apply it in the factory production line.

1 INTRODUCTION

In recent years, the optoelectronic industry has

flourished, and optoelectronic products have been

increasing day by day. The volume of optical

products continues to shrink, but the resolution

continues to improve. Therefore, the tolerance

requirements of optical components have become

more and more stringent. The problem of residual

stress in optical components has gradually attracted

attention. There will be residual stress during the

production, assembly and operation of optical

products The residual stress will directly affect the

accuracy and life of the product. Therefore, analyze

the internal stress of optical components and

eliminate residual stress has become the most

important problem in optical products.

Currently, the only way to measure the internal

stress state of a component is photoelastic

analysis(Holister, 1967). The measurement of

photoelastic is used to measure the stress and strain

on the surface or inside of the object by using the

polarization state of the light wave after passing

through the sample. In 1850 years, Maxwell proposed

Stress-optic Law and established the theoretical basis

Corresponding Author: bonochou@narlabs.org.tw

of photoelastic analysis(Aben etc., 2012). Many

researchers had published a lot of research on

photoelastic analysis technology based on this theory.

In 1979, Muller's team developed a fully automated

photoelastic analyzer using cameras and image

processing technology(Müller etc., 1979). The

principle was to capture five images from 0 degrees

to 90 degrees of isoclinic line and merged them into a

contour map. The contour map was used to determine

the internal stress distribution of the component. In

1986, Hecker's team developed a phase shift method

for stress measurement (Hecker etc., 1986). The

principle was to capture multiple images with

different angles of polarization. These pictures were

calculated by inverse trigonometric functions to

obtain isoclinic angle and fringe order. The

information of isoclinic angle and fringe order was

used to determine the internal stress distribution of

the component. In 1997, Petrucci's team combined

the phase shift method with color imaging

technology. It could reduce measurement errors

caused by wavelength (Petrucci etc., 1997). In 1998,

the Plouzennec team established a circularly

polarized light source photoelastic analysis system.

The system captured bright field and dark field

images to analysis the phase distribution and derived

106

Yang, C., Hsu, C., Chen, Y., Lin, Y., Tsai, H., Huang, K. and Chou, C.

Analysis of Optimal Polarization State Sampling Frequency Applied to Photoelastic Analyzer.

DOI: 10.5220/0010799900003121

In Proceedings of the 10th International Conference on Photonics, Optics and Laser Technology (PHOTOPTICS 2022), pages 106-110

ISBN: 978-989-758-554-8; ISSN: 2184-4364

the stress distribution from the phase

distribution(Plouzennec etc., 1999).

In the traditional stress measurement technologies

and equipment, it usually needed a long time and

high-precision calibration or expensive components.

Therefore, in the paper, we have established a

photoelastic analysis system, a measurement process

and a stress algorithm. The advantage of the system

was low cost and short measurement time. In the last

section of the paper, the photoelastic analysis system

was used to measure the sample stress distribution

with different sampling frequency. The different

sampling frequency data was used to build a chart

which was the relationship between measurement

error and sampling frequency. The chart could help

who used the method to choose a suitable sampling

frequency and establish the most efficient process of

stress measurement.

2 PHOELASTIC ANALYSIS

SYSTEM INTEGRATION

The photoelastic analysis system was mainly

composed of LED Light source, Linear polarizer, 1/4

waveplate, Band pass filter and imaging system. The

relative position of the element of the system was

shown in Fig 1. These elements were commercially

available. These elements didn’t require customized

production, which could reduce the cost of system

construction. The bill of material table was shown in

Table 1.

Table 1: The Photoelasticity bill of material table.

Photoelasticity BOM

Items

Discription

Light

source

Philips MR16 LED(4.2W) with

diffuser and collimator

Linear

polarizer

Visible Linear Polarizing Film

(

Over 99% Polarizin

Efficienc

1/4

waveplate

Polymer Achromatic Retarder

(630-835nm)

Band

pass filter

Transmission wavelength :

440-450 nm pass

Imaging

System

STC-MCS163U3V

 H x V pixels : 1440 x 1080

 Pixel pitch: 3.45 x 3.45 um

 Lens : M0814-MP2

In the system, there were several parameters that

must be paid attention to stability of output energy,

uniformity of spot irradiance, symmetrically of the

spot and the element relative levelness, when

assembling the system. In order to achieve high-

precision stress measurement, we formulate an

assembly and adjustment process, shown below:

 Element relative levelness: The illumination

surface of light source was regarded as

reference level. Each element level error should

less than 1 um.

 Stability of output energy: The visible light

sensor was used to measure the Light source

intensity error at different times. The Light

source intensity error should less than ± 1 uW

within 30 minutes.

 Symmetrically of the spot and uniformity of

spot irradiance: The symmetry and unformity

of the spot was directly related to the inclination

angle of the 1/4 waveplate. Therefore, fine-tune

the 1/4 wave plate until the light spot was

unformity and symmetrically distributed,

shown as Fig 2.

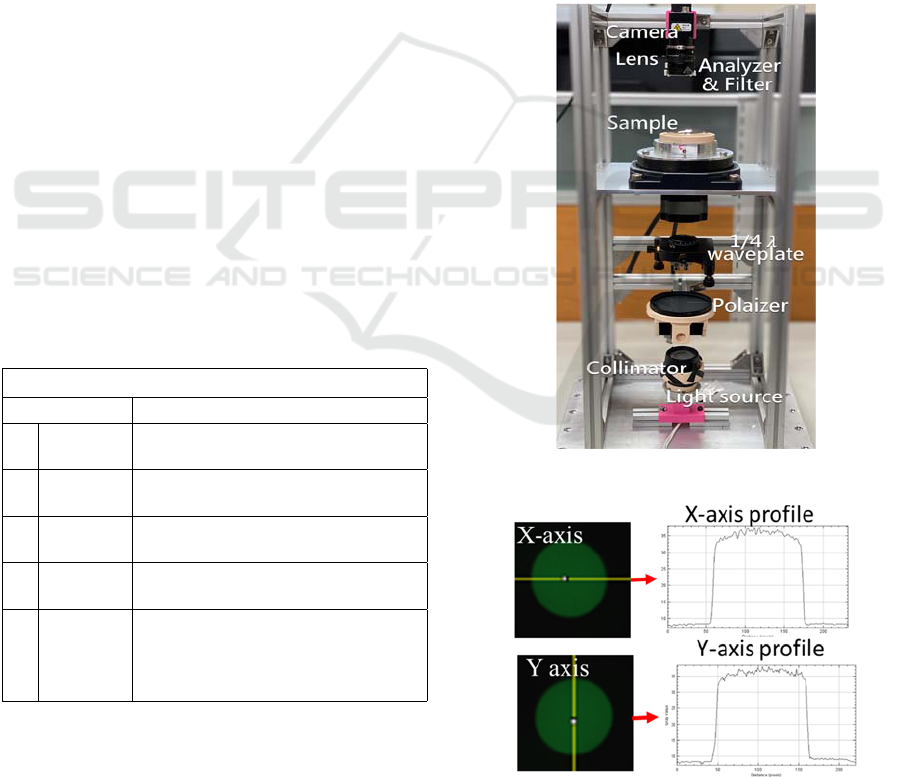
Figure 1: The photoelastic analysis system.

Figure 2: Analyze the symmetry and uniformity of the spot

by the cross-section of the spot.

Analysis of Optimal Polarization State Sampling Frequency Applied to Photoelastic Analyzer

107

 Checking the light source polarization state: If

the relative directionality of Linear polarizer

and 1/4 waveplate was incorrect, the light will

become elliptically polarized after passing

through the 1/4 waveplate. Therefore, it was

necessary to rotate the 1/4 waveplate so that its

direction corresponds to the Linear polarizer.

When the light source was circularly polarized,

the intensity captured by the sensor was equal,

even rotating the analyzer, shown as Fig 3.

Figure 3: The light Spot intensity cross-section profile at the

different angle of analyzer.

It was the photoelastic Analysis System assembly

and adjustment process. After completing the above

steps, you could start to measure the stress of the

sample.

3 STRESS CALCULUS

The stress distribution and the phase difference

distribution are in a proportional relationship. When

the relative phase shift was measured, the relative

stress distribution could be derived. Therefore, the

stress measurement system should focus on how to

obtain the phase shift quickly and accurately. For the

phase shift and stress distribution formula was shown

as Eq. (1)

δ





𝐶𝜎



𝜎



 (1)

Where 𝛅 is Phase retardation, t is Object Thickness,

C is Stress-optic coefficient and 𝜎

& 𝜎

is Principal

stresses.

In the paper, we used the phase shift method to

calculate stress. The principle was modulation the

input light wave into a circular polarization state and

analyze the polarization state of the light wave after

passing through the sample. The measurement

method of polarization state was to rotate analyzer

and capture the intensity of light spot at different

angle. According the different angle of sampling data,

the polarization state of each pixel could be derived,

shown as Fig 4.

When we got the polarization state of each pixel,

the phase shift could be derived. The formula was

shown below: Eq. (2)

𝑐𝑜𝑠φ 









∗





















∗



(2)

Where φ is the phase, ψ is the elliptical orientation

angle, E_x' is the maximum value of the E_x , E_y' is

the maximum value of the E_y.

Put the phase shift of each pixel into the Eq. (1)

and we could get the stress of each pixel. The process

of stress calculus was shown as Fig 5.

Figure 4: The method of polarization state calculus.

Figure 5: The process of stress calculation of photoelastic

analysis system.

4 STRESS MEASUREMENT AND

ANALYSIS

The previous section introduced the structure of the

photoelastic analysis system and stress calculation

PHOTOPTICS 2022 - 10th International Conference on Photonics, Optics and Laser Technology

108

method. The section presented the stress

measurement results and a average stress

measurement error chart. Here the doublet lens was

used as a sample. The sample diameter is 50 mm and

material is BK7. The material of glass lens does not

have a lot of stress. The stress in the doublet lens

mainly comes from the UV glue. In the experiment,

the” Norland Optical Adhesive 61” was used to

adhesive the double lens. The UV glue is cured by

ultraviolet light with maximum absorption within the

range of 320-380nm. The transmittance of UV glue is

over 90 % in the visible light. The UV glue refractive

index was 1.527 at liquid state. When the UV glue

was cured, the refractive index increased to 1.563.

Therefore, whether the glue was uniformly cured has

a great influence on the internal refractive index.

According to the optical theory proposed by Maxwell

in 1853, the change optical refractive index of a

transparent body was linearly proportional to the

stress. The relationship was as follows: Eq. (3)

𝑛



𝑛



𝐶𝜎



𝜎



 (3)

Where n

& n

is the refractive index of each

principal stress direction in the material, C is Stress-

optic coefficient and 𝜎

& 𝜎

is Principal stresses.

According the above formula, the internal stress

of the doublet lens will be affected by the uniformity

of illumination and the Curing time. The light source

used in this experiment was a self-made UV light

source. The irradiation area was about 4 inches,

Irradiance uniformity > 90% @ irradiation area and

the average of Irradiance was 5 mW/𝑐𝑚



. Before the

doublet glue lens was cured, the double glue lens

needed to go through the process of glue dispensing,

kneading and homogenization. Generally, the

thickness of the doublet lens adhesive was 50um. The

curing time usually needed 5 minute by self-made UV

light source. In this experiment, the standard samples

were produced according to the above-mentioned

conditions.

Currently, Commercial stress measurement

equipment available usually only measured

information from four angles, and fitted the

polarization state of each pixel through the

information of the four angles. Because there were

only four angle information, it was easy to cause

errors. The easiest way to overcome this problem was

to increase the sampling frequency, but frequent

sampling will cause the measurement time to be too

long. Therefore, this paper would conduct intensive

sampling analysis and provided an optimal sampling

frequency analysis based on the measurement results.

In this experiment, the sampling range was from

0 to 135 degrees and used 45 degrees, 22.5 degrees,

11.25 degrees and 5 degrees as the sampling

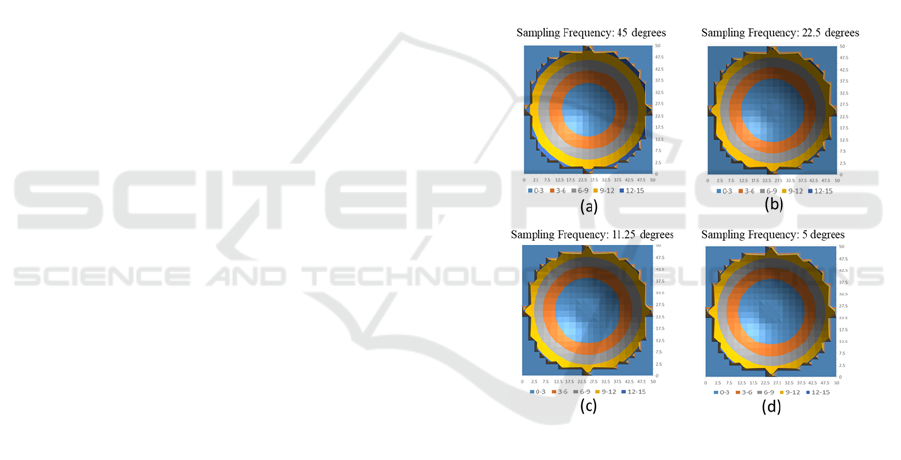
frequency. In the Fig 6, it shown the stress

distribution results at different sampling frequency.

For easy analysis, the X-axis cross-sectional profile

was obtained from the results of different sampling

frequencies for analysis, shown as Fig 7. Because the

5 degrees was highest sampling frequency, we

defined the measurement result of the 5 degrees

sampling frequency was the standard reference value.

According to the measurement results, when the

sampling frequency was higher, the measurement

error was smaller and closer to the reference value.

When the sampling angle was 11.25 degrees, the

stress cross section profile was almost overlaps with

the standard reference profile.

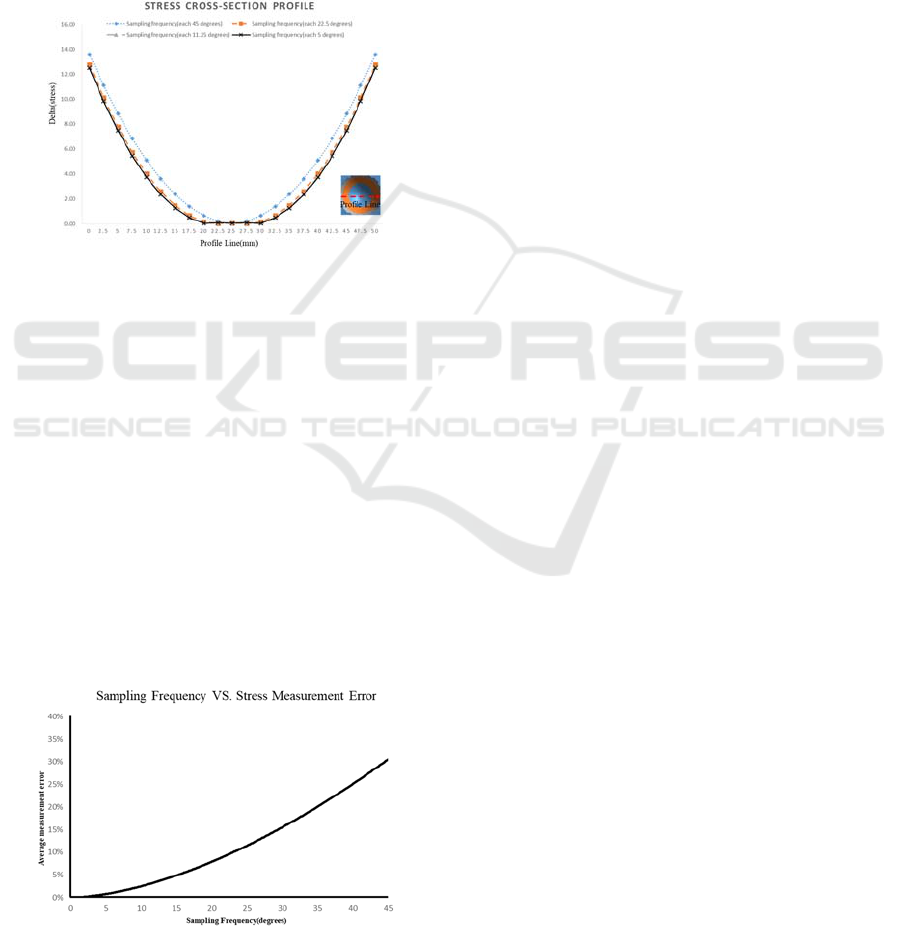
In order to obtain the parameters between

sampling frequency and measurement error, we used

the 5 degrees sampling angle as the standard

reference data, and calculated the average stress

measurement error with the results of other sampling

angles, shown as Fig 8. According the results, when

the sampling angle was less than 15 degrees, the

average stress measurement error was less than 5%.

Figure 6: The results of stress distribution at different

sampling frequency. (a) Sampling Frequency: 450. (b)

Sampling Frequency: 22.50. (c) Sampling Frequency:

11.250. (d) Sampling Frequency: 50.

5 DISCUSSION

In the doublet lens, the UV glue will generate stress

during the curing process. The stress will directly

affect the effective focal length of the doublet lens.

When the effective focal length changes, the optical

quality of the imaging system will also decrease.

Therefore, in the paper, we have established a

photoelastic analysis system, a measurement process

and a stress algorithm. The photoelastic analysis

system was used to measure the sample stress

Analysis of Optimal Polarization State Sampling Frequency Applied to Photoelastic Analyzer

109

distribution with different sampling frequency. The

different sampling frequency data was used to build a

chart which was the relationship between

measurement error and sampling frequency. The

chart could help who used the method to choose a

suitable sampling frequency. In this experiment, the

sampling range was from 0 to 135 degrees and used

45 degrees, 22.5 degrees, 11.25 degrees and 5 degrees

as the sampling frequency. Among them, the 5

degrees data was used to be standard reference data

and compared the reference data with the results of

other sampling frequencies.

Figure 7: The chart of cross-section profile of stress.

According to the measurement results, when the

sampling frequency was higher, the measurement

error was smaller and closer to the reference stress

distribution. When the sampling angle was 11.25

degrees, the stress cross section profile was almost

overlaps with the standard reference profile. In order

to obtain the parameters between sampling frequency

and measurement error, we used the 5 degrees

sampling angle as the standard reference data, and

calculated the average stress measurement error with

the results of other sampling angles. According the

results, when the sampling angle was less than 15

degrees, the average stress measurement error was

less than 5%. If people want to keep the measurement

speed and accuracy, the recommended sampling

frequency angle of photoelastic analysis system was

15 degrees.

Figure 8: The chart of average stress measurement error.

6 CONCLUSION

In the paper, we proposed a low-cost photoelastic

analysis system and used this system to measure the

stress distribution of the doublet lens. The doublet

lens was measured by four types of sampling

frequency. The sampling frequency was 45 degrees,

22.5degrees, 11.25degrees and 5degrees. We used the

measurement stress curve of 5 degrees to be a

reference. When the sampling frequency was

increased, the measure stress curve was closed to the

reference curve. Finally, we used the error of different

sampling frequency to build up a sampling frequency

and measurement error curve. Users could set the

sampling frequency according to their own

measurement accuracy requirements. In the future,

we hope to improve the photoelastic analysis system

to be fully automatic and apply it in the factory

production line.

ACKNOWLEDGEMENTS

The authors would like to express their appreciation

for financial aid from the Ministry of Science and

Technology. R.O.C, number MOST 109-2622-E-

492-011-CC3.

REFERENCES

GS Holister. (1967). Experimental stress analysis:

principles and methods. CUP Archive.

Aben, H., & Guillemet, C. (2012). Photoelasticity of glass.

Springer Science & Business Media.

Müller, R. K., & Saackel, L. R. (1979). Complete automatic

analysis of photoelastic fringes. Experimental

Mechanics, 19(7), 245-251.

Hecker, F. W., & Morche, B. (1986). Computer-aided

measurement of relative retardations in plane

photoelasticity. In Experimental stress analysis (pp.

535-542). Springer, Dordrecht.

Petrucci, G. (1997). Full-field automatic evaluation of an

isoclinic parameter in white light. Experimental

Mechanics, 37(4), 420-426.

Plouzennec, N., & Lagarde, A. (1999). Two-wavelength

method for full-field automated photoelasticity.

Experimental mechanics, 39(4), 274-277.

PHOTOPTICS 2022 - 10th International Conference on Photonics, Optics and Laser Technology

110