
Optimization of Emergency Medical Service with Fixed Centers
Marek Kvet
Faculty of Management Science and Informatics, University of Žilina, Slovakia
Univerzitná 8215/1, 010 26 Žilina, Slovakia
Keywords: Location Analysis, Urgent Healthcare System, Service Accessibility Optimization, Radial Approach,
Fixed Centers.
Abstract: The research reported in this scientific paper focuses on practical usage of optimization methods aimed at
improving the service accessibility for clients spread over the whole Slovak Republic. The results of previous
research confirmed by a computer simulation indicated that the weighted p-median problem is a suitable way
of optimization. Here, we pay attention to the inconvenience of current ambulance stations deployment, which
consists in the fact that there are same locations with two or more stations equipped with an ambulance vehicle.
On the other hand, the standard weighted p-median problem formulation allows locating at most one station
to one place. Furthermore, when searching for a better service center locations, the capacity of a center should
be taken into account at least partially. Otherwise, the station with a high number of assigned clients would
not be able to satisfy all the demands. Such result may be considered inacceptable. We believe that mentioned
disadvantages could be overcome by fixing some stations, which will not be allowed to change their current
location. The results of suggested optimization process are compared with the analysis of current ambulance
stations deployment form more points of view.
1 INTRODUCTION
Emergency Medical Service (EMS), fire brigades and
many other rescue systems are established to ensure
rapid information, activation and effective usage and
coordination of the forces and resources of rescue
services in providing the necessary assistance. The
role of such systems is to provide the affected person
with the necessary assistance in the case of a threat to
life, health or property without any delay to prevent
from irreversible losses on health or life. Obviously,
the quality and efficiency of the EMS system depends
mainly on the number of stations operating in the
considered area (in our case in the whole state) and
on the location of the stations. Determining the right
number of facilities is a very sensitive issue that must
take into account two conflicting requirements. The
first of them follows from the main mission of the
EMS system - to save the life and health of the
population. This task can be adequately fulfilled if the
network of EMS stations is dense enough. Then the
system is able to respond to an emergency call
immediately and can provide first aid in a short time.
On the other hand, there is a legitimate requirement
for the efficient use of public resources, which limits
the number of ambulance stations to be located.
Limiting the number of service providing facilities
results in an increase in their workload and a
reduction in the availability of emergency care, as the
nearest ambulance may be occupied at the time of an
emergency call by providing a service to another
patient. A situation in which a patient does not receive
urgent medical care within a predetermined time limit
is evaluated as a system failure (Brotcorne et al.,
2003, Current et al., 2002, Doernet et al., 2005,
Ingolfsson et al., 2008, Matiaško, Kvet, 2017).
Therefore, this paper focuses on the strategic level of
management of emergency health care. The main
attention is paid to determining the optimal locations
of EMS stations so that the accessibility of the service
for patients is the highest possible. It can be assumed
that the accessibility is the better the closer the EMS
station is to the affected patient (Jánošíková, 2007).
The reasons to optimize the EMS system (to find
new optimal service center deployment) may follow
from more ideas, not only from establishing a new
system. The necessity of system optimization usually
follows from the fact that the distribution of demands
changes in time and space. Naturally, the originally
determined stations deployment may not fit now.
214
Kvet, M.
Optimization of Emergency Medical Service with Fixed Centers.
DOI: 10.5220/0010890700003117
In Proceedings of the 11th International Conference on Operations Research and Enterprise Systems (ICORES 2022), pages 214-221
ISBN: 978-989-758-548-7; ISSN: 2184-4372
Copyright
c
2022 by SCITEPRESS – Science and Technology Publications, Lda. All rights reserved

Another reason for optimization of current EMS
system consists in basic performance characteristics.
Analysis of data given by EMS providers has shown
that the average response time, i.e. time necessary to
achieve the patient from the EMS station after an
emergency call, has risen by almost one minute in the
last years and thus the accessibility of urgent medical
care for patients in critical condition has worsened.
Such situation results not only in a deterioration in the
availability of the service, but possibly in an increase
in the number of unnecessary and avoidable deaths.
Furthermore, new roads have been built and the
traffic has changed, too. Therefore, the deployment of
current EMS stations should be regularly checked and
optimized if necessary. We believe that some changes
in the locations of EMS centers (without any change
of their number) may contribute to improve average
service accessibility for clients.
Let concentrate on the optimization process itself,
now. It must be realized that there are several cities or
smaller city districts, which are inhabited by a high
number of potential patients. Such big demands for
service are covered by more stations located at the
same address. Such a situation needs to be taken into
account also in the process of system optimization.
There are several ways to cope with this problem. If
we want to formulate a model that would allow
locating more stations at the same place, we should
follow the principle of multiple facility location
problem as suggested in (Janáček, 2021). In such a
case, the optimized design quality criterion should be
based on the concept of generalized disutility. It
means that the service does not have to be provided
only by the nearest located EMS station, but by the
nearest station being currently available (Kvet,
Janáček, 2018, Kvet et al., 2019). If the minimized
objective function takes into account only the
distance or travel time from clients’ locations to the
nearest located source of urgent healthcare, than the
mentioned modeling approach does not hold.
The optimization process studied in this paper is
based on two steps. In the first phase, some of EMS
stations get fixed. It means, they are not allowed to
change their current location due to the large number
of emergency calls assigned to them. The second
phase of the optimization approach is based on the
mathematical model, which searches for the best
possible locations of the remaining stations.
The structure of this paper is organized as follows.
The next section contains an analysis of current EMS
stations deployment and it explains the emergency
system in Slovakia. The third section contains the
details about the suggested optimization strategy and
the proposed mathematical model. In the fourth
section, we provide the readers with a computational
study, in which the results of suggested method are
presented. This section contains also a comparison of
the obtained results to the current state. Finally, the
last section brings some brief concluding remarks and
suggests new possible future research directions.
2 EMS SYSTEM IN SLOVAKIA
The Emergency Medical Service system represents a
pre-hospital part of the urgent care provision, which
forms the highest level of differentiated medical care.
It can be also defined as providing the urgent health
care to a person in such a condition in which their life
or health is suddenly endangered and the affected
person is dependent on the rescue service. The EMS
system is a part of the Integrated Rescue System of
the Slovak Republic.
In its current form, the EMS system in Slovakia
operates 274 stations, which can be divided according
to the type of crew into the following two groups:
1. RZP stations – The crew consists of two
members - a paramedic and an ambulance
driver, or two paramedics (one as a driver).
There are currently 188 ambulances of this
type in Slovakia. Some of them are equipped
with an incubator to transport newborns.
2. RLP stations – The ambulance staff consists
of three members: a doctor specialist in
emergency medicine, anesthesiology and
intensive care (or another specialization);
paramedic and an ambulance driver, or a
doctor with two paramedics. There are 86
such ambulances in the Slovak Republic.
In addition, the private company Air - Transport
Europe, operates 7 stations of the Helicopter Rescue
Medical Service. Some of the RLP ambulances are
equipped with a mobile intensive care unit for the
transport of critically ill patients. This special
equipment follows from the decision of the Ministry
of Health of the Slovak Republic based on the
recommendation of the Emergency Medical
Operations Center. In August 2014, the number of
extra equipped RLP stations was set at 5. Other types
of EMS ambulances, such as in the surrounding
countries, are not recognized by Slovak legislation
(Doerner et al., 2005, Marianov, Serra, 2002, Reuter-
Oppermann et al., 2017, Schneeberger et al., 2016).
For completeness, the RZP stations are located in
166 different places (in some of them, there are two
or even more). The RLP stations are placed totally in
80 locations. The total number of network nodes with
Optimization of Emergency Medical Service with Fixed Centers
215
at least one ambulance regardless of its type is 207.
These 274 stations cover the demands of totally 2,934
municipalities spread over the area of Slovakia.
As mentioned in previous parts of the paper, the
accessibility of the EMS is generally the better the
closer is the service provider to the client location.
From the point of the service access analysis, it is
necessary to distinguish two basic approaches:
1. Take into account the average distance of all
clients from the nearest station, regardless of
its type. In the case of selected specific
diagnoses from the first hour quintet, this
view may not be appropriate, because the
RLP needs to be present at the scene.
2. Analyze the distance only to the nearest RLP
station. This value of service accessibility
will be logically higher than in the previous
approach, but in cases of specific diagnoses,
it models the situation better.
The following Table I summarizes selected basic
performance characteristics of the system not only for
the entire system (RZP and RLP stations together),
but also for RLP type stations separately. Let us
remind that patients who need to be satisfied in the
case of an acute life and health threat are concentrated
in totally 2,934 municipalities. The municipality's
weight can be expressed in many different ways:
1. Number of emergency calls of patients in
critical or urgent condition (this number may
not be proportional to the population of the
municipality)
2. Absolute number of inhabitants sharing the
location
3. The value of one (all municipalities have the
same weight, the municipality size does not
play any role)
In this computational study, we report the results
for each possible weight of a client location. The final
selection of the correct municipality weight is up to
the decision-maker or other authority responsible for
the decision-making process. The basic numerical
characteristics to express the service accessibility are:
Average time in minutes the ambulance
vehicle needs to achieve the affected patient,
Maximal time in minutes that the ambulance
crew must travel to get to the farthest patient,
Percentage of demands covered within 8 or
15 minutes from the nearest located station.
The service providers defined the limits 8 and 15
minutes according to their internal rules following
from the standards of urgent healthcare.
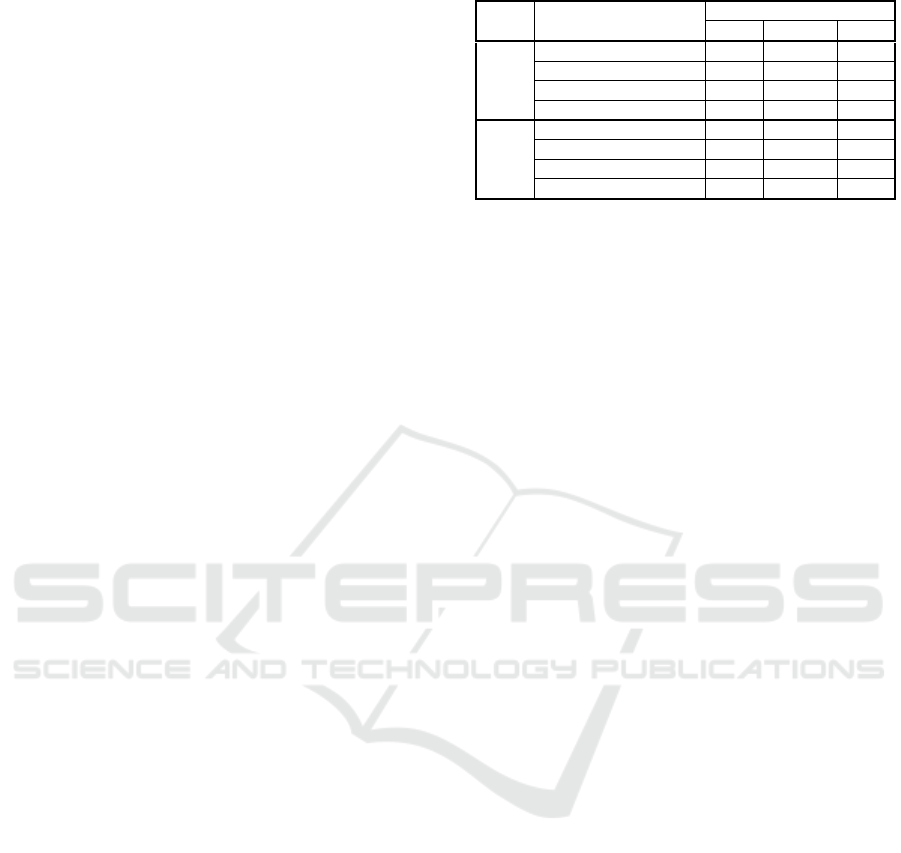
Table 1: Analysis of current EMS stations deployment.
Indicator
Municipality weight
Calls Citizens One
Entire
EMS
system
M
axima
l
response time 31.5 31.5 31.5
A
verage response time 5.73 5.72 7.75
15 min percentage 98.29 98.48 93.76
8 min
p
ercentage 73.28 73.03 48.06
RLP
only
M
aximal response time 38.1 38.1 38.1
A
verage response time 7.98 7.99 11.72
15 min percentage 86.75 87.09 69.97
8 min percentage 53.97 53.50 20.79
The analysis of current EMS stations deployment
shows many important things: The positive is that the
current situation is not bad, rather the opposite.
Almost 100 percent of requests can be satisfied within
15 minutes by the nearest located service center
regardless the type of ambulance staff. On the other
hand, the obtained characteristics indicate that the
accessibility of the service provided by RLP stations
only deserves a strong improvement. Therefore, this
research paper introduces an optimization method to
improve the current state. Presented approaches are
suggested in such a way that they perform only little
changes in current service center deployment with
great effect on service accessibility. We expect that
since the performed changes will not be too large,
public authorities responsible for EMS system
performance efficiency could accept them.
3 TWO-PHASE OPTIMIZATION
OF CURRENT EMS SYSTEM
The main goal of this section is to provide the readers
with the details of proposed optimization method
suggested to achieve better EMS stations deployment
mainly from the point of RLP stations performance
efficiency.
As it was mentioned in the introducing section,
the proposed optimization method is based on two
phases. Since we want to make such a mathematical
model, which avoids locating more than one center to
the same place, and we assume the objective function
to consider only the nearest EMS station for each
client, the first phase of the algorithm consists in pre-
processing those center locations, in which there are
currently more than one facility.
The first phase does not contain any optimization.
Its core idea consists in pre-processing the input data.
Let us introduce necessary denotations to formulate
the first phase precisely. We will use the set I to
denote the set of locations, which are the candidates
for ambulance station locating. Similarly, the symbol
J will denote the set of served municipalities.
ICORES 2022 - 11th International Conference on Operations Research and Enterprise Systems
216

Obviously, the sets I and J can be the same as it is in
our case. Each element jJ is connected with its
weight denoted by w
j
, which is usually an integer or
real number. The coefficient w
j
can be interpreted in
many different ways. As already mentioned, it can be
the number of expected emergency calls from the
location j, it can express the number of inhabitants
sharing the location j, etc. In our implementation of
the algorithm, the weight w
j
represents the number of
calls of patients in a critical or urgent condition. The
operators of current EMS stations provided us with
the weights. Since the first phase may decrease the
original values of w
j
, we will use the symbol c
j
to
denote the final weight of a municipality j used in the
next steps of the optimization process. In many cases,
c
j
= w
j
, but there will be also some locations, for
which c
j
< w
j
or even c
j
= 0. If c
j
= 0 then all demands
of the municipality jJ are covered by the stations
located at j, which are not allowed to change their
location. Furthermore, it can be generally assumed
that an ambulance regardless of its type has limited
capacity and it is able to serve only Q = 19919 calls.
This value is the average number of calls assigned to
one station. If a station must remain in its current
location, it is excluded from further optimization. At
the same time the weight w
j
of given municipality j is
reduced by Q in such a way that it can not drop below
zero. If we denote the current number of stations in
municipality j by the symbol r
j
, then three different
situations may occur:
1. If w
j
> r
j
Q, the stations cannot be relocated.
The uncovered demand in municipality j
that represents an input parameter of the
model is c
j
= w
j
− r
j
Q.
2. If w
j
> Q and at the same time w
j
< r
j
Q, then
/
j
wQ
stations must remain in the
municipality, the others may be relocated.
The uncovered demand in municipality j
will be c
j
= w
j
mod Q.
3. If w
j
< Q and r
j
> 1, one station must
remain in the town, the others may be
relocated. Municipality j is completely
served by the fixed station and so the
uncovered demand will be c
j
= 0.
The last rule is related to managerial decisions
made in the past. There are not apparent reasons why
there are multiple stations in some small towns today
(maybe a good hospital is nearby). Nevertheless, we
try to respect them to some extent since severe
changes in the current system may not be acceptable.
This way, we get the list of stations, which must
stay at their current place and new values of the
weights c
j
. After the fixation of some centers in their
current location, the type of stations must be decided
about. We prefer fixing the RLP stations. If there are
more stations that need to be fixed, we fix the RLP
stations at the particular location first (if there are
any) and then we add the remaining number of RZP
stations. This first phase results in 48 fixed RLP and
19 fixed RZP stations according to the rules 1 and 2.
The stations, which should be fixed according to the
rule 3 are not added to the list of fixed station due to
the following fact. The capacity of the previously
fixed stations is fully utilized and the stations fixed
according to the rule 3 have some free capacity to
accept additional calls. Therefore, for each station
fulfilling the rule 3 we add a separate constraint in the
next step in order to keep the station located at its
current place, but we allow to assign some additional
calls to it. Let all such stations form the set F.
After obtaining the list of fixed centers and the list
of stations for which we need additional constraint in
the following mathematical model, we can formulate
the second step of suggested optimization method.
All centers, which are not fixed, should undergo an
optimization process in order to find the best location
to achieve the optimal value of used criterion. Since
we have two types of EMS stations, first, we will find
the best locations of the stations regardless their type,
and then we will decide about the type of stations.
To formulate the mathematical model, we need to
introduce
further notation. As above, let the symbol I
denote the set of candidates for EMS station locating.
In our case, the set I contains all 2,934 cities and
villages of Slovakia. The same elements are used to
form the set J of used municipalities. As a weight of
individual location jJ we use the coefficient c
j
obtained from the first phase. As far as the objective
function is concerned, it considers the average time
the ambulance vehicle from the nearest center needs
to achieve the affected patient. Let the time distance
between the locations iI and jJ be denoted by t
ij
.
Finally, we need the integer number p of stations to
be located. In our case, p = 274 – 48 – 19 = 207. To
complete the model, the decision about locating a
EMS station at the location iI will be modelled by a
binary variable y
i
{0, 1}. The location-allocation
formulation of the model does not hold because of the
model size (the sets I and J contain approximately
3,000 elements each) and the necessity to model
assignment of clients to their located centers. The
matrix of allocation variables would contain about 9
million variables (Avella et al., 2007). To overcome
this obstacle, so-called radial formulation can be used
(Avella et al., 2007, García et al., 2011, Janáček,
2008, Kvet, 2014, Kvet, 2015).
Optimization of Emergency Medical Service with Fixed Centers
217

In accordance with the radial formulation, let the
symbol m denote the largest value in the matrix {t
ij
},
i.e. m = max{t
ij
: iI, jJ}. For simplicity, we assume
that all values in the matrix are integer. Of course, the
radial formulation can be easily adjusted also for real
values. For each location jJ and for each integer
value v = 0, 1 … m-1 we introduce a variable x
jv
{0,
1}. This variable takes the value of one, if the time t
j*
spent by travelling from the client located at jJ to
the nearest EMS station is greater than the value of v
and it takes the value of zero otherwise. Then, the
expression (1) holds for each jJ.
1
*
0
m
jjv
v
tx
(1)
Similarly to the set-covering problem, a binary
matrix {a
s
ij
} must be computed according to the
formula (2), in which iI, jJ and v = 0, 1 … m-1.
1if
0otherwise
ij
v
ij
tv
a
(2)
After these preliminaries, the radial model of the
problem can be formulated by the expressions (3)-(8).
1
0
m
j
jv
jJ v
M
inimize c x
(3)
:1
,0,1 1
v
jv ij i
iI
Subject to x a y
for j J v , ,m
(4)
i
iI
yp
(5)
1
f
yforfF
(6)
{0, 1}
i
yforiI
(7)
{0, 1} , 0, 1 1
jv
xforjJv,,m
(8)
The quality criterion of the design formulated by
the objective function (3) expresses the sum of time
distances from all clients to their nearest EMS station.
The link-up constraints (4) ensure that the variables
x
jv
are allowed to take the value of 0, if there is at least
one center located in radius v from the location j and
the constraint (5) limits the number of located EMS
stations by
p. The constraint (6) follows from the first
phase of suggested approach and its task is to arrange
that the centers fixed according to the rule 3 stay at
their current locations. The last obligatory constraints
(7) and (8) keep the domain of the variables
y
i
and x
jv
.
It must be realized that the optimal solution of the
model (3)-(8) may bring such system design that
differ from the current stations deployment a lot. If
the proposed changes are too large, then they do not
have to be accepted neither by private EMS providers
nor by public authorities responsible for the service.
Therefore, we suggest a simple model extension,
which would limit the number of current stations,
which can change their location. Such an extension is
seemingly simple, but it can be achieved only with
additional constraint. The problem is to define a
change in center locating. Generally, a change is
performed only in such a case, if there is a location,
in which more centers will be located than there are
currently. Therefore, we need to introduce a constant
n
i
for each iI. This constant takes the value of one,
either if all EMS stations at the location
i are fixed or
if there is no station located at
i. In case when only
some of the current stations are fixed, but not all of
them, this coefficient takes the value of zero. Then the
model (3)-(8) can be extended by the expression (9),
in which the parameter
q limits the number of stations
that are allowed to change their current location. The
value of
q is the parameter of suggested method.
ii
iI
ny q
(9)
By solving the model (3)-(9) we obtain the list of
the optimal locations of EMS stations regardless of
their types. The final decision about the type of each
located ambulance can be made in a simple way. Let
all EMS stations following from the result of the
model (3)-(9) form a set of candidates for further
processing. The model (3)-(8) can be used again to
select the RLP stations from the set of all located
centers. Obviously, the input data need to be adjusted.
To sum up the whole optimization approach, it is
based on two phases. First, the biggest cities are
solved and if there are more EMS stations located at
the same place, some of them get fixed according to
the defined rules. The stations which do not get fixed
are subject of the optimization, which consists of two
steps. In the first one, we find the optimal locations of
all EMS station and then, we select the RLP stations
from the set of all candidates.
4 COMPUTATIONAL STUDY
The main goal of performed computational study is to
show and compare the obtained results for various
settings of parameter
q to the current EMS stations
deployment. The results are summarized on the
following Tables 2-7. Each table contains the results
of different value of
q. Note that the parameter q
expresses the maximal number of centers, which can
change their current location.
ICORES 2022 - 11th International Conference on Operations Research and Enterprise Systems
218
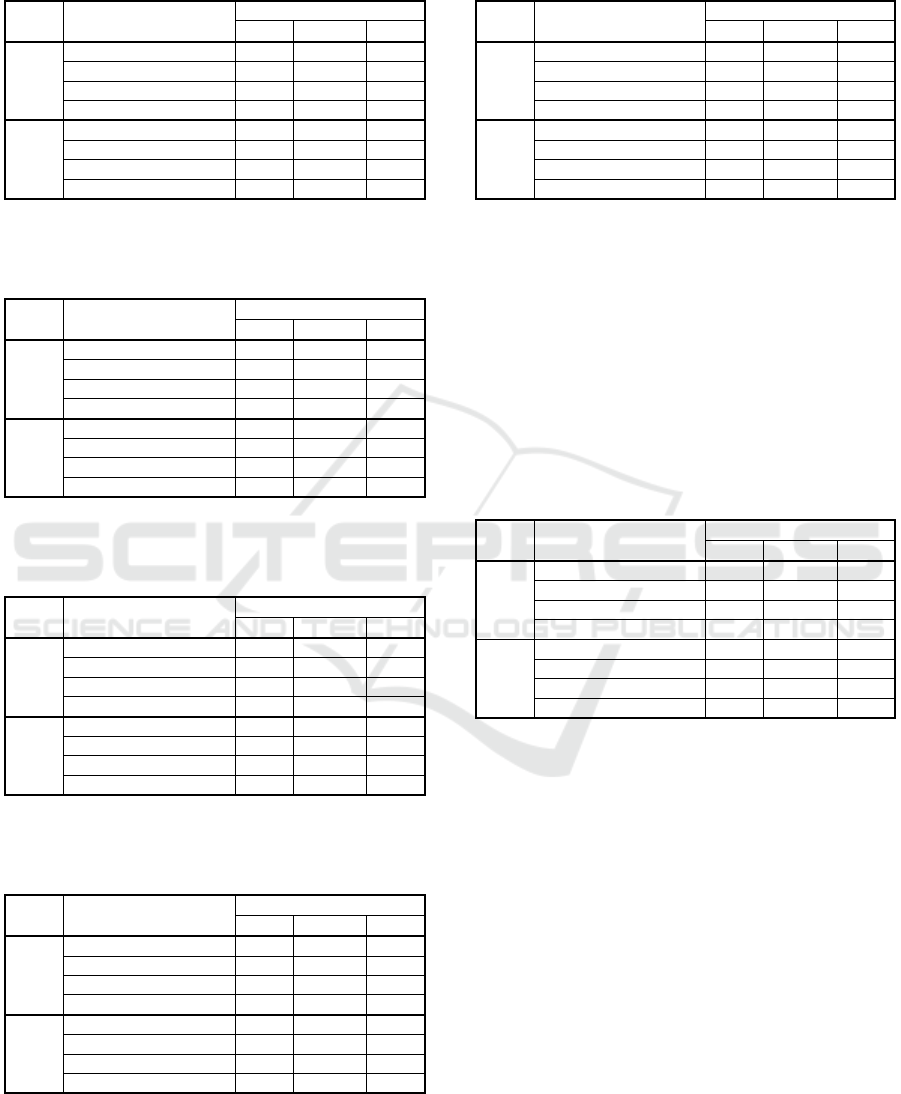
Table 2: Analysis of EMS system design obtained by
suggested optimization approach, in which at most 10
percent of current EMS stations are allowed to be relocated.
Indicator
Municipality weight
Calls Citizens One
Entire
EMS
system
M
aximal response time 30.2 30.2 30.2
A
verage response time 5.28 5.36 7.41
15 min percentage 98.71 98.82 94.51
8 min percentage 77.94 76.69 52.15
RLP
only
M
aximal response time 38.1 38.1 38.1
A
verage response time 7.75 7.82 11.43
15 min percentage 88.84 88.81 71.98
8 min percentage 54.52 53.55 21.57
Table 3: Analysis of EMS system design obtained by
suggested optimization approach, in which at most 20
percent of current EMS stations are allowed to be relocated.
Indicator
Municipality weight
Calls Citizens One
Entire
EMS
system
M
aximal response time 25.4 25.4 25.4
A
verage response time 5.04 5.12 7.27
15 min percentage 98.83 98.98 94.61
8 min percentage 82.30 80.84 53.99
RLP
only
M
aximal response time 38.1 38.1 38.1
A
verage response time 7.73 7.78 11.44
15 min percentage 88.72 88.80 72.02
8 min percentage 55.20 54.26 21.85
Table 4: Analysis of EMS system design obtained by
suggested optimization approach, in which at most 30
percent of current EMS stations are allowed to be relocated.
Indicator
Municipality weight
Calls Citizens One
Entire
EMS
system
M
aximal response time 25.4 25.4 25.4
A
verage response time 4.92 5.02 7.13
15 min percentage 99.02 99.12 95.13
8 min percentage 83.07 81.48 55.08
RLP
only
M
aximal response time 38.1 38.1 38.1
A
verage response time 7.73 7.78 11.43
15 min percentage 88.59 88.71 71.88
8 min percentage 55.07 54.19 22.02
Table 5: Analysis of EMS system design obtained by
suggested optimization approach, in which at most 40
percent of current EMS stations are allowed to be relocated.
Indicator
Municipality weight
Calls Citizens One
Entire
EMS
system
M
aximal response time 25.1 25.1 25.1
A
verage response time 4.83 4.94 7.04
15 min percentage 99.04 99.13 95.33
8 min percentage 83.77 82.09 55.79
RLP
only
M
aximal response time 38.1 38.1 38.1
A
verage response time 7.73 7.79 11.43
15 min percentage 88.47 88.56 72.09
8 min percentage 55.16 54.06 22.05
Table 6: Analysis of EMS system design obtained by
suggested optimization approach, in which at most 50
percent of current EMS stations are allowed to be relocated.
Indicator
Municipality weight
Calls Citizens One
Entire
EMS
system
M
aximal response time 25.1 25.1 25.1
A
verage response time 4.77 4.88 6.98
15 min percentage 99.15 99.24 95.54
8 min percentage 84.28 82.68 56.75
RLP
only
M
aximal response time 38.1 38.1 38.1
A
verage response time 7.73 7.80 11.48
15 min percentage 88.21 88.33 71.47
8 min percentage 54.89 53.90 22.09
The last Table 7 reports the results of suggested
optimization approach, in which the number of
centers allowed to be relocated does not play any role.
It means that the parameter
q was set to the value of
p. All centers could change their current locations,
except of those being fixed. This way, the additional
constraint (9) does not make any sense and it can be
excluded from the model. Table 7 keeps the structure
of previously reported tables.
Table 7: Analysis of EMS system design obtained by
suggested optimization approach, in which all current EMS
stations except for the fixed are allowed to be relocated.
Indicator
Municipality weight
Calls Citizens One
Entire
EMS
system
M
aximal response time 25.1 25.1 25.1
A
verage response time 4.76 4.87 6.97
15 min percentage 99.11 99.22 95.57
8 min percentage 84.21 82.64 56.51
RLP
only
M
aximal response time 38.1 38.1 38.1
A
verage response time 7.74 7.81 11.50
15 min
p
ercentage 88.15 88.27 71.44
8 min percentage 54.75 53.79 22.05
The results reported in Tables 2-7 show that the
higher the number of stations allowed to be relocated,
the better service accessibility can be achieved. In
other words, the mathematical model respecting
given limitations tries to locate the center in those
places, in which they are the nearest to the demand
points. On the other hand, if we look at the results for
RLP stations only, the obtained results indicate that
the coverage within the time limit of 15 minutes is
quite good, but the coverage by the time of 8 minutes
is still insufficiently weak. This observation deserves
development of another approach aimed primarily at
optimization of RLP stations.
As far as the computational time requirements of
suggested approach are concerned, the computational
times are not reported here. It must be noted that the
first phase does not contain any optimization process
and the fixation of EMS stations can be computed via
one cycle very fast. The second phase consists in
Optimization of Emergency Medical Service with Fixed Centers
219

solving the above-described model (3)-(9) to obtain
the optimal locations of all EMS stations and then, the
model (3)-(8) is used again to select the RLP stations.
The radial formulation makes the model simpler than
the location-allocation formulation and thus, the
optimal solution of the problem can be reached by
about three minutes using a common notebook with
standard technical parameters and basic equipment.
5 CONCLUSIONS
This paper was focused on practical usage of the
optimization method based on the weighted
p-median
problem formulation. The goal of optimization was to
achieve better access to urgent medical healthcare
provided by private or public emergency agencies.
Suggested method was based on current station
deployment analysis, which showed that there are
some station locations with multiple facilities. This
fact should be considered also in the optimization
process. Such a request may cause several difficulties
when formulating the model. The researchers could
either create a model with multiple facility locations
and apply the concept of generalized disutility or this
obstacle could be handled in a different way.
The optimization approach studied in this paper is
based on two phases. The first phase searches for such
stations, that can not change their current locations for
different reasons. After that, a simple model based on
the weighted
p-median problem is solved to obtain
the optimal location of EMS stations. All located
EMS stations become candidates for RLP, which are
searched for by solving another mathematical model.
Since the radial formulation enables us to solve real-
world sized instances, we hope that suggested method
can significantly contribute to the state-of-the-art in
the field of service system optimization approaches.
Obviously, this method is not the only possible way
to improve current stations deployment.
Based on achieved results reported in previous
section, the future research in this scientific are could
be aimed at RLP stations, which could be primarily
solved by the second phase of suggested algorithm.
Another scientific topic to be studied could be focus
on developing new algorithms, which would be able
to apply different criteria and bring better results.
ACKNOWLEDGEMENT
This work was supported by the research grant VEGA
1/0216/21 “Design of emergency systems with
conflicting criteria using artificial intelligence tools”.
This work was supported also by the Slovak Research
and Development Agency under the Contract no.
APVV-19-0441.
REFERENCES
Avella, P., Sassano, A., Vasil’ev, I., 2007. Computational
study of large scale p-median problems. In
Mathematical Programming, Vol. 109, No 1, pp. 89-
114.
Brotcorne, L., Laporte, G., Semet, F., 2003. Ambulance
location and relocation models. European Journal of
Operational Research 147, pp. 451–463.
Current, J., Daskin, M., Schilling, D., 2002. Discrete
network location models. In Drezner Z. (ed) et al.
Facility location. Applications and theory, Berlin,
Springer, pp. 81-118.
Doerner, K. F. et al., 2005. Heuristic solution of an
extended double-coverage ambulance location problem
for Austria. In Central European Journal of Operations
Research, Vol. 13, No 4, pp. 325-340.
García, S., Labbé, M., Marín, A., 2011. Solving large p-
median problems with a radius formulation. INFORMS
Journal on Computing, Vol. 23, No 4, pp. 546-556.
Ingolfsson, A., Budge, S., Erkut, E., 2008. Optimal
ambulance location with random delays and travel
times, In Health Care Management Science, Vol. 11,
No 3, pp. 262-274.
Janáček, J., 2008. Approximate Covering Models of
Location Problems. In Lecture Notes in Management
Science: Proceedings of the 1st International
Conference ICAOR, Yerevan, Armenia, pp. 53-61.
Janáček, J., 2021. Multiple p-Facility Location Problem
with Randomly Emerging Demands. In: Proceedings of
the 14th International Conference on Strategic
Management and its support by Information Systems
2021, pp. 120-125.
Jánošíková, Ľ., 2007. Emergency Medical Service
Planning. Communications Scientific Letters of the
University of Žilina, 9(2), pp. 64-68.
Kvet, M., 2014. Computational Study of Radial Approach
to Public Service System Design with Generalized
Utility. Digital Technologies 2014: Proceedings of the
10th Interna-tional IEEE Conference, pp. 198-208.
Kvet, M., 2015. Advanced Radial Approach to Resource
Location Problems. Studies in Computational
Intelligence: Developments and Advances in Intelligent
Systems and Applications, Springer, pp. 29-48.
Kvet, M., Janáček, J., 2018. Reengineering of the
Emergency Service System under Generalized
Disutility. In the7th International Conference on
Operations Research and Enterprise Systems ICORES
2018, Madeira, Portugal, pp. 85-93.
Kvet, M., Janáček, J., Kvet, M., 2019. Computational Study
of Emergency Service System Reengineering Under
Generalized Disutility. Operations Research and
Enterprise Systems: 7th International Conference,
ICORES 2022 - 11th International Conference on Operations Research and Enterprise Systems
220

ICORES 2018 Funchal, Madeira, Portugal, January 24–
26, 2018, Springer, pp. 198-219.
Marianov, V., Serra, D. 2002. Location Problems in the
Public Sector. Drezner, Z. (Ed.): Fa-cility location -
Applications and theory, Berlin: Springer, pp. 119-150.
Matiaško, K., Kvet, M. 2017. Medical data management.
Informatics 2017: IEEE International Scientific
Conference on Informatics, Danvers: Institute of
Electrical and Electronics Engineers, pp. 253-257.
Reuter-Oppermann, M., van den Berg, P. L., Vile, J. L.
2017. Logistics for Emergency Medical Service
systems. Health Systems, Vol. 6, No 3, pp 187-208.
Schneeberger, K. et al. 2016. Ambulance location and
relocation models in a crisis. Central European Journal of
Operations Research, Vol. 24, No. 1, Springer, pp. 1-27.
Optimization of Emergency Medical Service with Fixed Centers
221
