Multi-view NURBS Volume

Wanwan Li

Department of Computer Science

George Mason University, Fairfax, VA, U.S.A.

Keywords:

Geometric Modeling, Interactive Interface, Numerical Optimization, NURBS Volume.

Abstract:

Non-Uniform Rational B-Spline (NURBS) curves and surfaces are widely used in modern geometric model-

ing systems. NURBS volumes, also called volumetric NURBS, are one powerful NURBS representation of

volumetric modeling. However, due to the complex nature of NURBS volumes, it is a challenging task for

users to ﬁne-tune the NURBS volumes design manually. In this paper, we present a novel approach for multi-

view NURBS volume geometric modeling. Given users’ conceptual design for several different views of a

3D model, we devise an optimization algorithm to automatically reconstruct the 3D NURBS volume which is

matching with these designs by projecting it along with different view directions. In the end, we discuss the

results generated with our approach through a series of numerical experiments.

1 INTRODUCTION

Non-Uniform Rational B-Spline (NURBS) is a math-

ematical formulation that represents the controllable

geometry of curves and surfaces in 2D or 3D space.

NURBS curves and surfaces can be precisely created

and edited by users through control points. There-

fore, modern 3D modeling software such as Autodesk

3Ds Max, Maya, Blender, SketchUp, Revit, Rhino,

Camera 4D, etc. all support the input as NURBS ge-

ometry. Due to the controllability of NURBS curves

and surfaces, they are widely used in digital art de-

sign, industrial design, architectural design, game de-

sign, and animation movie industry, etc. There are

a great number of researches on user interface stud-

ies for computer-aided designs (CAD) that are mostly

focused on the NURBS curves and surfaces for devel-

oping smarter interfaces of 2D or 3D modelings.

However, as another natural extension of NURBS

curves and surfaces, NURBS volumes design is an-

other interesting topic to explore. NURBS volumes

are scalar ﬁelds who are consisting of a couple of

scalar numbers. Those scalar numbers are controlled

by some control values. Volumetric data are com-

mon in the data processing and data visualization

community. Especially, volumetric data are often

used in medical science, boolean operations, and

points clouds computing, etc. Therefore, volumet-

ric NURBS have great potential to be connected with

volumetric data processing and volumetric 3D mod-

eling directly. Unfortunately, due to the complexity

of controlling the NURBS volumes and large com-

putation complexity, the application of volumetric

NURBS has not been widely studied so far and it still

remains an open challenge to design a smart interface

for controllable NURBS volume design. Given these

observations, we devise the ﬁrst user controllable in-

terface for smart volumetric NURBS design. Through

our approach presented in this paper, users can design

their expected NURBS volumes through digital de-

signs on three different views, front view, left view,

and top view. As shown in Figure 1 (a), given these

input from an arbitrary device (in this case, a cell

phone), we visualize them onto three orthogonal walls

in Unity3D (b); and optimize the NURBS volume so

that it can automatically match with three-views after

being projected along three-axis (c). The main contri-

butions of our work include:

• Opening an interesting topic about design-driven

multi-view NURBS volume modeling.

• Devising an optimization approach to automati-

cally ﬁne-tune the NURBS volumes to match with

users’ input of designs on different views.

• Conducting a series of numerical experiments to

validate our approach and discuss its limitations.

2 RELATED WORK

NURBS Geometry Reconstruction. Using NURBS-

based geometry to reconstruct objects’ 2D proﬁle

curves or 3D manifold surfaces is a popular re-

search topic. Since the late 20th century, (Lavoie

et al., 1999), camera views-based NURBS shapes

228

Li, W.

Multi-view NURBS Volume.

DOI: 10.5220/0010850200003124

In Proceedings of the 17th International Joint Conference on Computer Vision, Imaging and Computer Graphics Theory and Applications (VISIGRAPP 2022) - Volume 1: GRAPP, pages

228-235

ISBN: 978-989-758-555-5; ISSN: 2184-4321

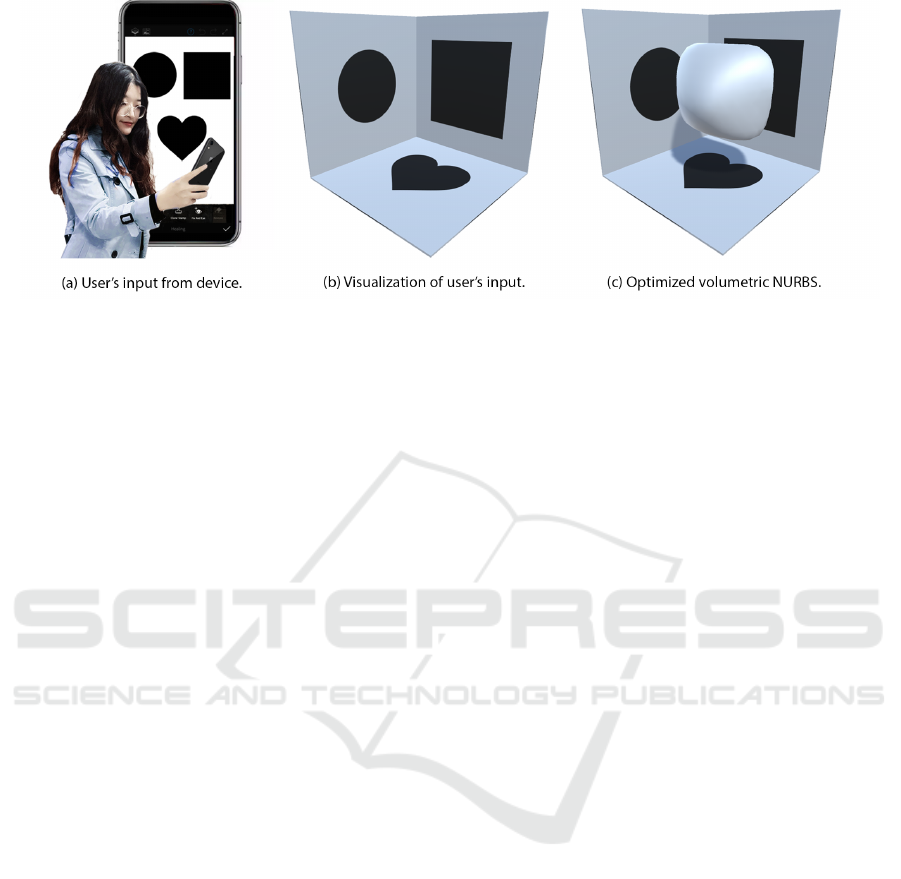
Figure 1: Demo of multi-view volumetric NURBS design. (a) Given the user’s digital painting of shapes projected from three

view directions including the front, top, and left view, (b) The User’s three-view design is visualized on three walls in the

Unity3D interface. (c) Finally, we optimize the NURBS volume to match with such a design from the three view directions.

modeling has been widely studied. For example,

a high-precision technique for reconstructing three-

dimensional (3D) objects from its one-camera 2D

views and using a color-coded structured light is de-

scribed by Lavoie et al. (Lavoie et al., 1999). In their

approach, the lines extracted from a view of the ob-

ject are used for constructing the NURBS curves so

as to generate the surface of the object. In 2008, Pal

et al. (Pal, 2008) proposed a reconstruction method

using geometric subdivision and NURBS interpola-

tion to successfully bridge the geometric subdivi-

sion and NURBS reconstruction on subdivided data,

NURBS patch and topology planning, construction

of trimmed NURBS surfaces, and writing IGES of

result patches. At the same time, the NURBS ge-

ometry model has been applied in digital terrain re-

construction for hydropower engineering based on

the TIN model proposed by Zhong et al. (Zhong

et al., 2008). In 2012, Imani et al. (Imani and

Hashemian, 2012) reconstructed the 2D proﬁles us-

ing a constrained local ﬁtting algorithm to approx-

imate the points segments through NURBS curves

with geometric continuity conditions. Later, in 2018,

Hashemian et al. (Hashemian and Hosseini, 2018)

propose an integrated approach for ﬁtting and fair-

ing smooth NURBS curves and surfaces simultane-

ously to reconstruct 3D objects elegantly through op-

timizations. At the same time, the article proposed

by (Vu-Bac et al., 2018) presents a gradient-based op-

timization algorithm to recover the applied loads and

deformations of thin shell structures through NURBS

geometry. NURBS curves can also be applied in tra-

jectory reconstruction for robot programming (Ale-

otti et al., 2005). Multi-view-based reconstruction

on NURBS curves has been successfully explored by

Saini et al. (Saini et al., 2015). In their project, two

perspective images deﬁning the curves are used as the

input and a nonlinear optimization process is used to

ﬁt a NURBS curve on the projected curves in these

images. Later, the same group (Saini et al., 2021)

proposed a two-view-based free-form NURBS curve

shape reconstruction algorithm using the Generalized

Ant Colony Optimizer (GACO) model. However,

none of the existing work has explored using NURBS

volumes to represent the 3D shapes of users’ geomet-

ric modelings and designs. Therefore, in this paper,

we explore the possibility and capability of interac-

tive controllable NURBS volume modeling.

Multi-view Geometric Modeling. Besides those

works on multi-view NURBS curves reconstructions

mentioned earlier, there are lots of other works

on multi-view geometric modeling. In computer

stereo vision, calibration images are mostly used for

3D shape reconstruction. For example, Sinha et

al. (Sinha and Pollefeys, 2005) describes a novel ap-

proach for reconstructing a closed continuous sur-

face from multiple calibrated color images and sil-

houettes by strictly enforces silhouette constraints

and optimize photo consistency with smoothness at

the same time. Labatut et al. (Labatut et al., 2007)

proposed approach focused on large-scale cluttered

scenes under uncontrolled imaging conditions. Later,

Fuhrmann et al. (Fuhrmann et al., 2014) proposed

MVE, an aulti-view reconstruction environment that

takes into consideration the structure-from-motion

(SfM) techniques, depth maps estimation using multi-

view stereo (MVS), and colored mesh extraction us-

ing a surface reconstruction approach called FSSR.

The work most closely related to our work is the

framework for multi-view wire art design proposed by

Hsiao et al. (Hsiao et al., 2018). In this work, an op-

timization approach is devised to optimize a wire art

design which can be projected along different view

directions to generate different sketchings that look

the same as the users’ inputs. This work inspires us

to devise an optimization algorithm to synthesize a

Multi-view NURBS Volume

229

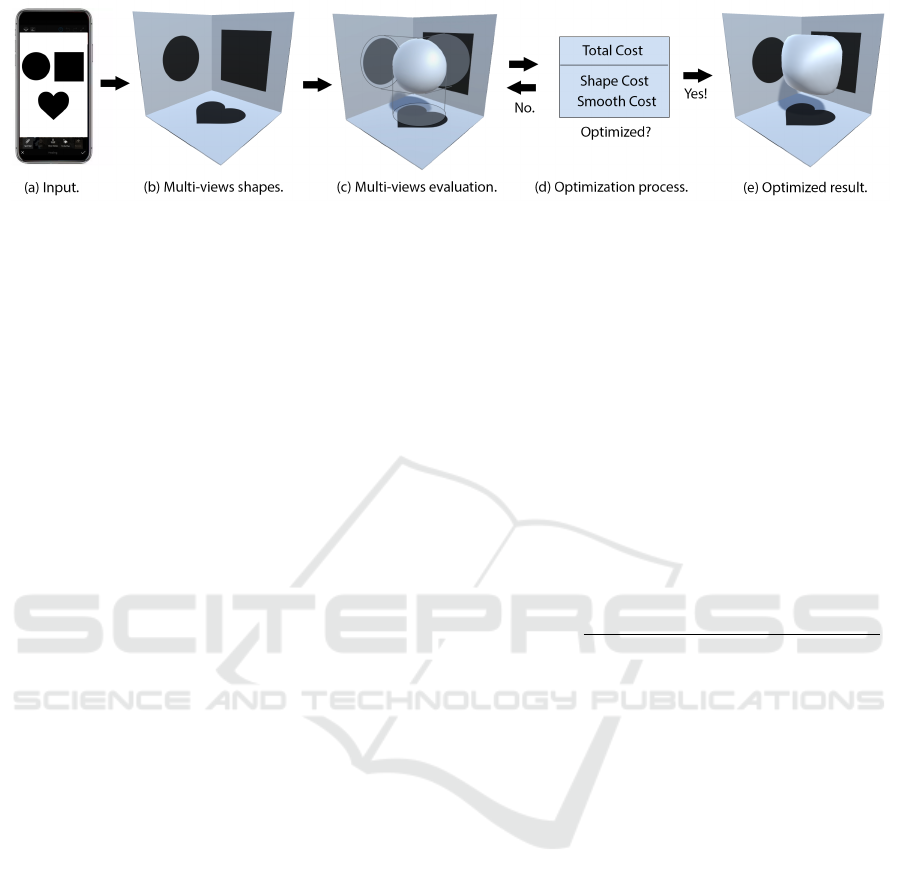
Figure 2: Overview ot our approach.

NURBS volume that can automatically match with

users’ inputs in a similar way.

3 OVERVIEW

Figure 2 shows the overview of our approach. (a)

Given the user’s input as the shapes deﬁned from

three views, in this case, the user paints three dif-

ferent shapes through an app on a mobile device.

(b) we convert the shape images to binary images

and project them onto three different walls within a

3D scene modeled in Unity3D. (c) We initialize the

NURBS volume with control values of zeros and con-

trol weights of ones. We extract the NURBS isosur-

face with an isovalue of 0.5. Then, we evaluate the

correctness of the NURBS volume shape by project-

ing it onto three different views and compare them

with the user’s input shapes respectively, projected

shadows plotted as gray circle areas. (d) During the

optimization process, we consider two important as-

pects of the NURBS volume shape, the ﬁrst is shape

cost which measures the difference between the gen-

erated NURBS volume and the user’s input according

to the evaluation process shown in (c). The second

is the smoothness of the generated NURBS volume,

as we hope it can be as smooth as possible. Without

this regularization term of smoothness cost, the re-

sulted NURBS volume will become rough and wrin-

kled so that the generated surface is not acceptable.

We will compare and discuss the effects of generated

NURBS isosurface with and without smooth costs

in the later section. For each optimization step, we

check whether the generated NURBS volume is op-

timized according to two considerations: (1) whether

the maximum number of iteration is reached and (2)

whether the total cost value is not changing which

means a local minimum is reached. If the result is

not optimized, we update the control values and con-

trol weights of the NURBS volume and go back to the

evaluation step shown in (c); Otherwise, we output the

ﬁnal optimized result as shown in (e). As we can see

from the result shown in this example, the generated

NURBS volume has a shape that looks like the user’s

input where the front is a square, the left is a circle and

the top is a heart. The result isosurface of optimized

NURBS volume is a smooth manifold surface.

4 PROBLEM FORMULATION

NURBS Volume. We formulate the NURBS volume

as a continuous scalar ﬁeld deﬁned through m × n × l

control values V

i, j,k

and control weights W

i, j,k

, where

i ∈ [1,m], j ∈ [1,n], and k ∈ [1,l]. Then the scalar

ﬁeld f (u,v, w) of NURBS volume at arbitrary position

(u,v,w) ∈ R

in the parameter space is calculated as:

f (u,v,w) =

∑

i, j,k

(u)B

(v)B

(w)W

i, j,k

∑

(u)B

(v)B

(w)W

i, j,k

where u,v, w ∈ [0,1] and B

(u), B

(v), B

(w) are p,

q, r-order B-spline base function at i, j, k-th control

values respectively. For simplicity, in this paper, we

use the same order for p, q, r as the control order o

for the NURBS volume. By default, we set o = 3.

NURBS Isosurface. We extract the isosurface of

NURBS volume given an arbitrary isovalue C such

that f (u,v,w) = C using a matching cube algorithm.

The isosurface is the result manifold surface that rep-

resents the NURBS volume. By default, we set the

isovalue C = 0.5. In the later section, we will change

the isovalue to discuss the differences in the synthe-

sized results. Our goal of the problem formulation is

to ﬁnd an isosurface such that it can match with the

user’s input from different views as much as possible.

Total Cost Function. We formulate this problem as

an optimization problem to ﬁnd the NURBS volume

scalar ﬁeld function f

∗

(u,v,w) calculated by control

values V

∗

and control weights W

∗

that can minimize

the total cost function C

total

( f ) which is deﬁned as:

total

( f ) = w

shape

( f ) + w

smooth

( f ), (1)

where C

shape

( f ) is the shape cost function of NURBS

volume f and C

smooth

( f ) is the smooth cost func-

tion of NURBS volume f . w

shape

and w

smooth

are the

GRAPP 2022 - 17th International Conference on Computer Graphics Theory and Applications

230

(a) 5

iteration. (b) 10

iteration. (c) 20

iteration. (d) 50

iteration. (e) 100

iteration.

Figure 3: Optimization process. Black shapes which are mapped onto the 3D walls are designed by the user. Figure (a-

d) shows the intermediate results generated through the optimization process. During the process, as the cost values are

decreasing, the isosurface of the NURBS volume is improving progressively to match with the user’s design. Figure (e)

shows the result of the ﬁnally optimized NURBS volume. As we can see, the result is similar to the user’s input.

weights set for shape cost and smooth cost respec-

tively. We empirically set a larger smooth cost weight

smooth

= 0.9 and smaller shape cost w

shape

= 0.1 for

achieving a better result. More details of shape cost

and smooth cost are discussed in later subsections.

Shape Cost Function. As one of the most impor-

tant costs for shape matching optimization, the shape

cost function is always used for evaluating how many

differences there are between the synthesized shape

and the target shape. Let 2D binary scalar ﬁeld func-

tion x(u, v), y(w,u), and z(w, v) are interpolated from

the binary shape images deﬁned by the user for top

view, left view and front view respectively. Noted

that after the 2D interpolation, these binary scalar

ﬁelds parameter space (u,v, w) are consistent with the

NURBS volume scalar ﬁeld function f (u,v, w) that

we want to optimize. As we want the optimized

NURBS volume to satisfy all three views, we need

to use multiplication to achieve the effects of Boolean

AND operation. Therefore, we calculate the shape

cost through the volumetric integral of the differ-

ence between the multiplication of the binary shapes

g(u,v,w) and the NURBS volume f (u, v,w) within the

parameter space. The shape cost is calculated as:

shape

( f ) =

ZZZ

|g(u,v,w) − f (u,v,w)|

dudvdw,

(2)

where g(u,v, w) = x(u,v)y(w,u)z(w, v) and the volu-

metric parameters u,v,w ∈ [0,1].

Smooth Cost Function. In order to generate a

smooth volumetric NURBS ﬁeld, we penalize the

rapid changes that happened to the volumetric ﬁeld

in the nearby area at an arbitrary position. Here,

we introduced the Laplace operators (∇

) to calcu-

late the sharpness of volumetric NURBS scalar ﬁeld

f (u,v,w). By minimizing the volumetric integral of

the divergence (∇·) of the gradient (∇) of the volu-

metric NURBS scalar ﬁeld, we will have the entire

volumetric ﬁeld as smooth as possible. The smooth

cost C

smooth

is represented as:

smooth

( f ) =

ZZZ

∇

f (u,v,w)dudvdw, (3)

where the volumetric parameters u,v, w ∈ [0,1] and

the Laplace operator (∇

) for three dimensions co-

ordinates (u,v,w) is given by the sum of the second

partial derivatives for both u, v, and w which is:

∇

f (u,v,w) =

∂

∂u

∂

∂v

∂

∂w

(4)

Optimization Process. We use L-BFGS (Limited-

memory BFGS) (Liu and Nocedal, 1989) to op-

timize the NURBS volume. L-BFGS is an

optimization algorithm in the family of quasi-

Newton methods that approximates the Broy-

den–Fletcher–Goldfarb–Shanno algorithm (BFGS)

using a limited amount of computer memory. Ac-

cording to the total cost function of the NURBS vol-

ume C

total

( f ) described in Equation 1. L-BFGS can

automatically ﬁnd the gradient of the total cost func-

tion ∇C

total

( f ) and update those control values and

control weights according to the gradients. Repeating

this process until the maximum number of iterations

is reached, the NURBS volume is optimized. In L-

BFGS, for each iteration step, there is an approximate

Hessian matrix and a search direction solved from the

gradient. Iteration step size is solved through a one-

dimensional optimization (line search), then the up-

dates are the search direction multiplied by the step

size. These optimization steps are applied several

times until a local minimal solution is found or the

maximum number of iterations is reached.

As shown in Figure 3, the NURBS volume is ﬁne-

tuned automatically with an L-BFGS optimizer. Dur-

ing the optimization process, the control values and

control weights of the NURBS volume are corrected

step by step through the L-BFGS optimization algo-

rithm without any user interactions. As we can see

from the subﬁgures, the optimization algorithm con-

verges pretty fast and get quite satisfying result within

Multi-view NURBS Volume

231

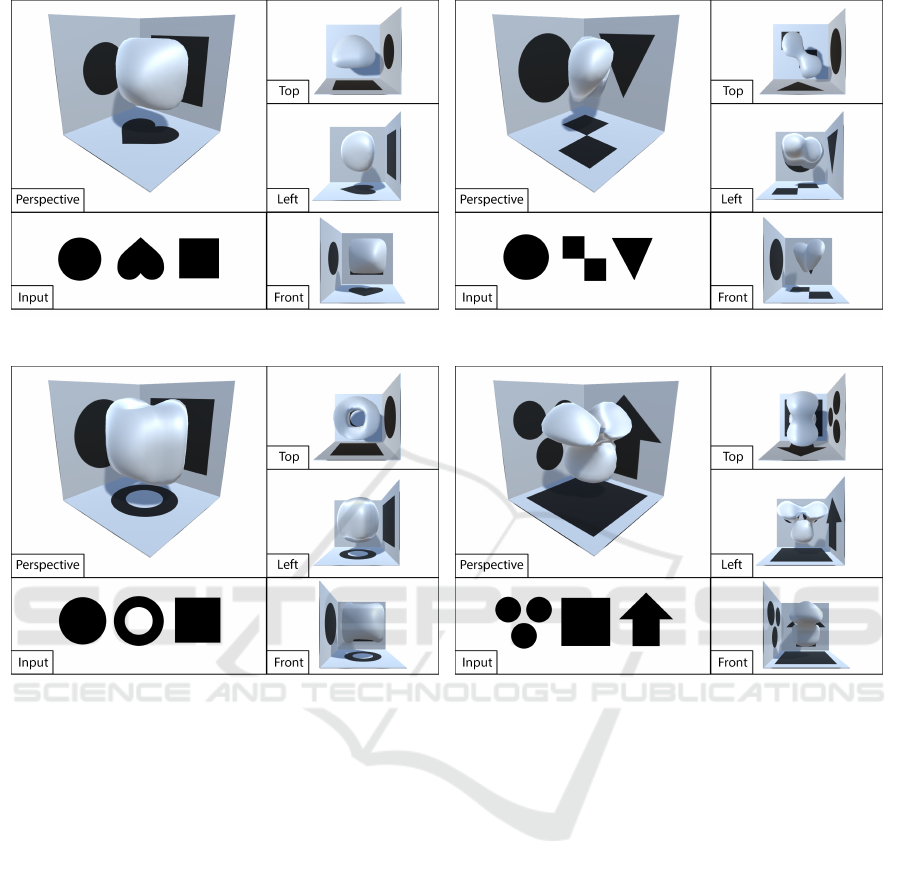
(a) User input 1: circle, heart, and square. (b) User input 2: circle, blocks, and triangle.

Figure 4: Experimental results of multi-view volumetric NURBS generated with different user inputs. In this ﬁgure, we

present the visual effects when applying different types of user input of shapes that are deﬁned for different view directions. In

subﬁgure (a-d), they present four different volumetric NURBS isosurfaces generated with our proposed optimization approach

according to four different user inputs. As we can see, most results can match the input shapes on different views accordingly.

the ﬁrst 50 iterations. In this example, we set the max-

imum number of iterations as 100 and the result is vi-

sually acceptable in the end.

5 EXPERIMENTAL RESULTS

Implementations. We have implemented the pro-

posed multi-view volumetric NURBS design inter-

face using Unity 3D with the 2019 version. Our

proposed L-BFGS optimization algorithm is imple-

mented in MinGW C++ using the StanMath and

Eigen library. The hardware conﬁgurations contain

Intel Core i5 CPU, 32GB DDR4 RAM, and NVIDIA

GeForce GTX 1650 4GB GDDR6 Graphics Card.

Changing User Inputs. We tested our optimiza-

tion approach on generating multi-view volumetric

NURBS with respect to different user inputs. As

shown in Figure 4, four different volumetric NURBS

isosurfaces are generated with our proposed optimiza-

tion approach according to four different user inputs.

All examples are generated with same parameter set-

tings: control order o = 3, ctrl size m = n = l = 50,

cell size is 20 × 20 × 20 and the max optimization it-

erations are 100. For each iteration, it typically takes

about 4 sec. Overall, all of the examples are typically

generated with about 400 sec. Those isosurfaces ex-

tracted from the optimized NURBS volumes in these

examples are set up with an isovalue of C = 0.5.

Generally speaking, most of the results demon-

strated here are seeming to match with the user’s in-

put shapes from different view directions. For exam-

ple, the front view in (a) looks like a square exactly,

the front view in (b) looks pretty much like a trian-

gle, the top view in (c) looks like a circle as is ex-

pected, and the left view in (d) shows three dots there

GRAPP 2022 - 17th International Conference on Computer Graphics Theory and Applications

232

Figure 5: Experimental results of changing isovalues. In this ﬁgure, we present the visual effects of applying different

isovalues when extracting isosurfaces on the same optimized NURBS volumes. Four different rows present four different

volumetric NURBS optimized from the four user inputs speciﬁed in Figure 4 with the parameters settings claimed earlier.

Four different columns demonstrate four different isosurfaces for each NURBS volume given four isovalues settings, they

are C =0.2, 0.4, 0.6, and 0.8 respectively. As we can see there is a trend that as the isovalue C grows higher, the shape of

isosurfaces shrinks. Therefore, we need to ﬁnd a balance point to best describe the optimized NURBS volume. According to

the empirical experiences, typically C ∈ [4.5,5.5] results in better visual effects.

clearly. But there are some imperfections in some

view directions such as the left view in (a) and (b)

look not like a perfect circle and the top view in (d)

is not a square and the front view in (d) does not look

like an arrow. This can be explained as we hope to

ensure the smoothness in the generated NURBS vol-

umes, therefore, low-level details such as sharp edges

on the arrow will disappear automatically. We will

discuss more about how to improve the result’s qual-

ity through precision control in a later experiment.

Changing Isovalues. We conducted numerical exper-

iments to test the visual effects of changing isovalue C

when extracting the isosurface in optimized NURBS

volume ﬁelds f

∗

(u,v,w) = C. As shown in Figure 5,

different isovalue results in different isosurfaces re-

sults. In overall, there is a trend that higher isovalue

results in a thinner shape of isosurfaces. For example,

as shown in the last example in the ﬁgure, when we set

Multi-view NURBS Volume

233

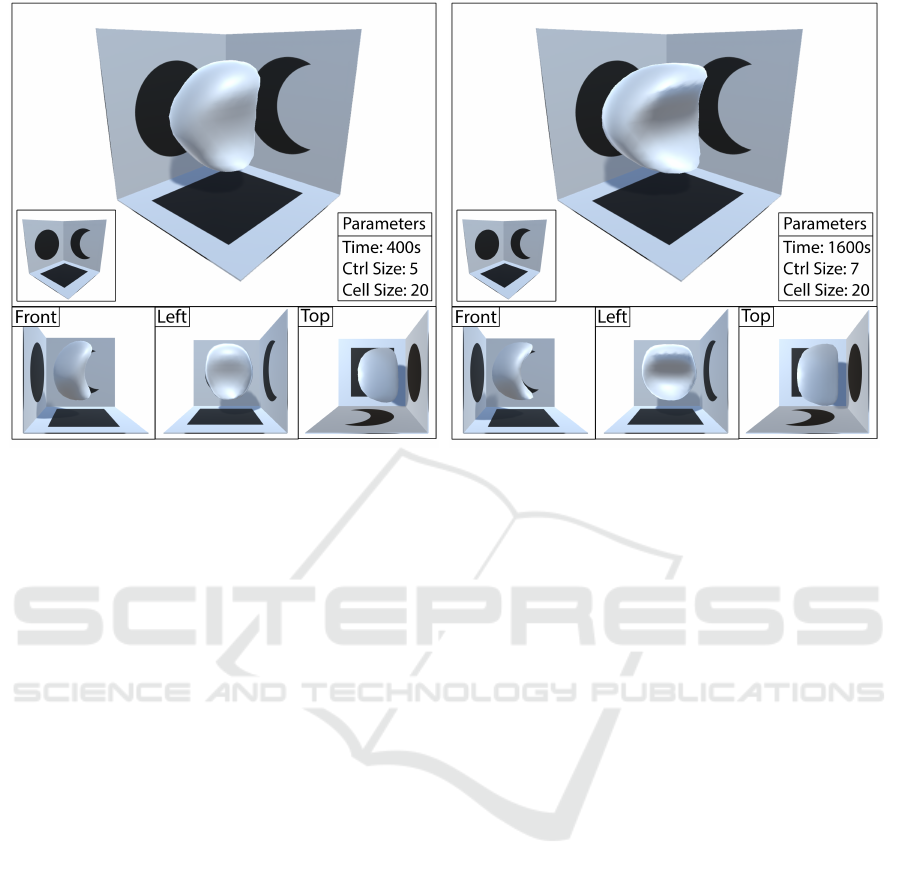
(a) Lower control precision. (b) Higher control precision.

Figure 6: Experimental results of improving control precision. This ﬁgure shows a comparison result between (a) lower

control precision and (b) higher control precision. In (a) the control size is m = n = l = 5 while in (b) the control size is

m = n = l = 7. Both result isosurfaces are extracted with the same cell size 20 × 20 × 20 and same isovalue C = 0.5. As we

can see there are differences in the detail of the shape between different levels of precision control. In (b) with higher control

degrees, the shape of the moon in the front view matches better than (a) which is generated with lower control degrees.

C = 0.2, the isosurface is too fat to see three dots any-

more, but when we set C = 0.8 the isosurface is too

skinny so that there are only three dots (spheres) left.

Most experiments’ results follow the rule that mod-

erate isovalues settings result in acceptable visual ef-

fects that are neither too fat nor too skinny. Typically

a balance point C ∈ [4.5,5.5] can best describe the op-

timized NURBS volume which are matching with the

user’s input shapes pretty well.

Improving Control Precision. As pointed out ear-

lier, low precision control over the optimized NURBS

volume will cause a loss of low-level details in the re-

sulting isosurface such as the sharp edges deﬁned in

the shapes drawn by users. Here we discuss more de-

tails about improving the control precision. As shown

in Figure 6, results on two different levels of con-

trol precision are presented. (a) shows a result with

a smaller precision control size which is 5. While

(b) shows a higher precision control size which is 7.

Therefore, the difference between the two control lev-

els are 7

−5

= 218 and there is a 218/5

= 74% in-

crease over the control degree. As we can see, higher

control precision results in an isosurface with more

levels of geometric details. For example, from the

front view, (a) shows a moon-like shape that is fatter

in middle and there is no sharp corner at two end-

ing points. But from (b), we can see a more realistic

moon shape which has a thinner middle part but with

sharper corners at two ends. We appreciate such an

improvement in the quality of the result, but the price

is the three times more seconds spent on optimizing a

NURBS volume with higher control precision.

Missing Smoothness Control. In our proposed opti-

mization algorithm, by default, we enforce a smooth-

ness consideration on the optimized NURBS vol-

umes. Let us explore what happens if we turn off the

smooth cost and ignore the smoothness control. As

shown in Figure 7, (a) a rough isosurface extracted

from the NURBS volume optimized without smooth

cost and (b) a smooth isosurface extracted from the

NURBS volume optimized with smooth costs turned

on are presented. As we’d prefer to generate smoother

results, therefore, the smooth cost is a very important

factor need to be considered during the optimization.

Failure Cases. During the experiments, we realize

there are lots of settings that result in failure cases.

First, the control size can not be too small, e.g., 1, 2,

3, ... In these cases, the NURBS volume can not be

optimized at all and results in some random NURBS

values. It also fails when cell size is too high, say, 25

In this case, no isosurface can be extracted from the

NURBS volume. If the control size is too large, say

m = n = l = 10, no enough memory space is available

for such a high space-demanding computational task.

GRAPP 2022 - 17th International Conference on Computer Graphics Theory and Applications

234

Figure 7: Experimental results of missing smoothness control. This ﬁgure shows a comparison result between (a) without

smooth cost and (b) with smooth cost. Both in (a) and (b) shares the same control size of m = n = l = 5 and their result

isosurfaces are extracted with the same cell size 20 × 20 × 20 and same isovalue C = 0.5. As we can see, (a) shows a rough

surface where there are lots of abrupt changes along the edges in the NURBS volume, while (b) shows a smooth surface where

the NURBS volume has smoother distributions over its NURBS ﬁeld.

6 CONCLUSIONS

In this paper, we present a novel interface for multi-

view NURBS volume geometric modeling. We de-

vise an optimization algorithm to automatically re-

construct the 3D NURBS volume which is match-

ing with user’s designs from different view directions.

Through a series of results, we show that our proposed

approach can reconstruct the NURBS volume that

matches with the user’s designs. At the same time,

we conclude that moderate isovalue settings, smooth-

ness considerations, and higher control precision will

result in better results. But higher precision needs ex-

tra time for optimization. We believe our work can

inspire more follow-up research to explore how the

volumetric NURBS design can change the industry

of the CAD and graphics industry.

REFERENCES

Aleotti, J., Caselli, S., and Maccherozzi, G. (2005). Tra-

jectory reconstruction with nurbs curves for robot

programming by demonstration. In 2005 Interna-

tional Symposium on Computational Intelligence in

Robotics and Automation, pages 73–78. IEEE.

Fuhrmann, S., Langguth, F., and Goesele, M. (2014). Mve-

a multi-view reconstruction environment. In GCH,

pages 11–18. Citeseer.

Hashemian, A. and Hosseini, S. F. (2018). An integrated

ﬁtting and fairing approach for object reconstruction

using smooth nurbs curves and surfaces. Computers

& Mathematics with Applications, 76(7):1555–1575.

Hsiao, K.-W., Huang, J.-B., and Chu, H.-K. (2018). Multi-

view wire art. ACM Trans. Graph., 37(6):242–1.

Imani, B. and Hashemian, S. A. (2012). Nurbs-based

proﬁle reconstruction using constrained ﬁtting tech-

niques. Journal of Mechanics, 28(3):407–412.

Labatut, P., Pons, J.-P., and Keriven, R. (2007). Efﬁcient

multi-view reconstruction of large-scale scenes using

interest points, delaunay triangulation and graph cuts.

In 2007 IEEE 11th international conference on com-

puter vision, pages 1–8. IEEE.

Lavoie, P., Ionescu, D., and Petriu, E. (1999). A high pre-

cision 3d object reconstruction method using a color

coded grid and nurbs. In Proceedings 10th Interna-

tional Conference on Image Analysis and Processing,

pages 370–375. IEEE.

Liu, D. C. and Nocedal, J. (1989). On the limited memory

bfgs method for large scale optimization. Mathemati-

cal programming, 45(1):503–528.

Pal, P. (2008). A reconstruction method using geomet-

ric subdivision and nurbs interpolation. The interna-

tional journal of advanced manufacturing technology,

38(3):296–308.

Saini, D., Kumar, S., and Gulati, T. R. (2015). Reconstruc-

tion of free-form space curves using nurbs-snakes and

a quadratic programming approach. Computer Aided

Geometric Design, 33:30–45.

Saini, D., Kumar, S., Singh, M. K., and Ali, M. (2021).

Two view nurbs reconstruction based on gaco model.

Complex & Intelligent Systems, pages 1–18.

Sinha, S. N. and Pollefeys, M. (2005). Multi-view recon-

struction using photo-consistency and exact silhou-

ette constraints: A maximum-ﬂow formulation. In

Tenth IEEE International Conference on Computer

Vision (ICCV’05) Volume 1, volume 1, pages 349–

356. IEEE.

Vu-Bac, N., Duong, T. X., Lahmer, T., Zhuang, X., Sauer,

R. A., Park, H., and Rabczuk, T. (2018). A nurbs-

based inverse analysis for reconstruction of nonlinear

deformations of thin shell structures. Computer Meth-

ods in Applied Mechanics and Engineering, 331:427–

455.

Zhong, D., Liu, J., Li, M., and Hao, C. (2008). Nurbs recon-

struction of digital terrain for hydropower engineer-

ing based on tin model. Progress in Natural Science,

18(11):1409–1415.

Multi-view NURBS Volume

235